Center for Fundamental Physics (CFP)
With Tabletop Experiments
Ulrich Jentschura at the Missouri University of Science and Technology
Higher-Order Corrections to Quantum Cyclotron Levels and Environment-Induced Corrections
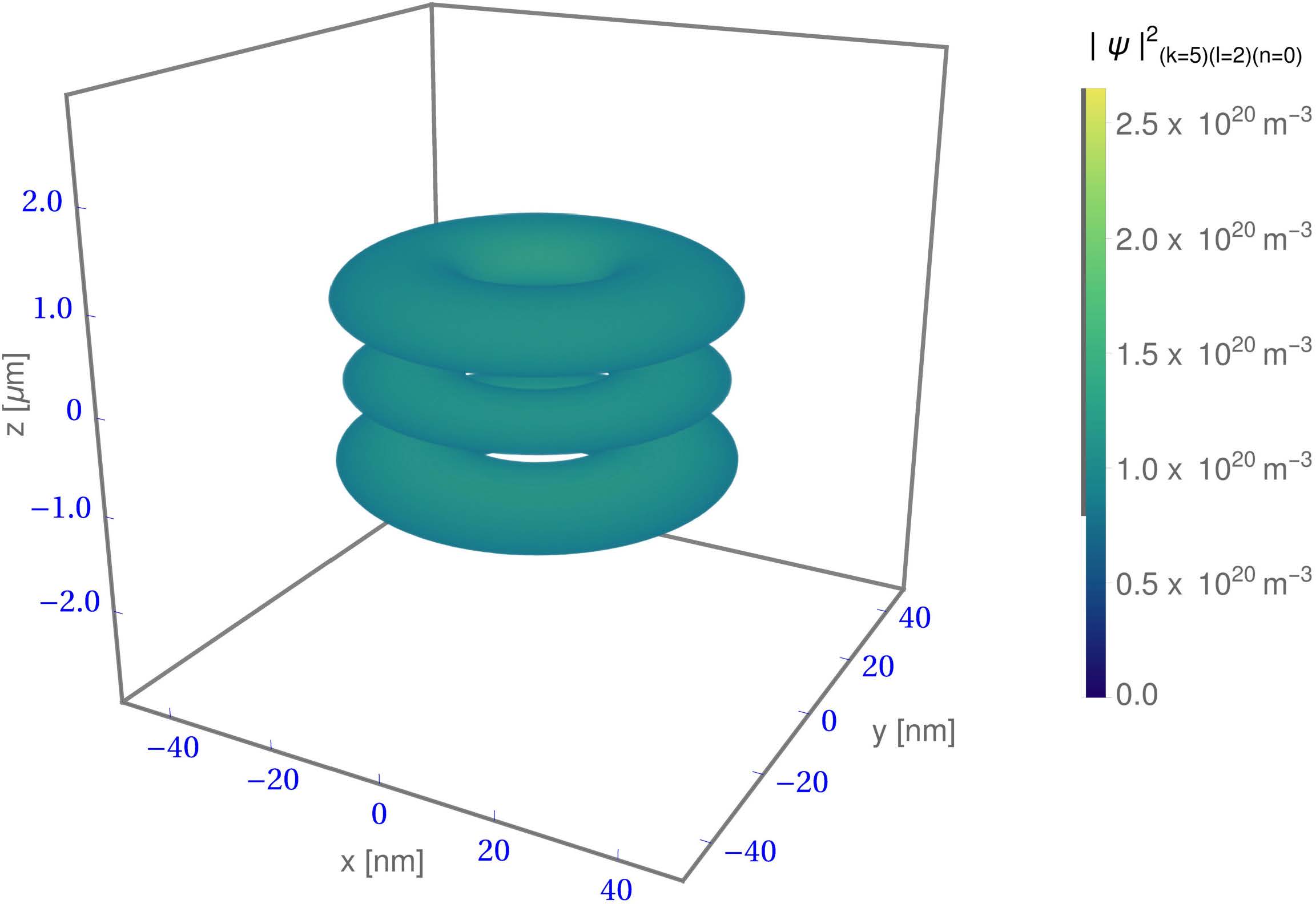
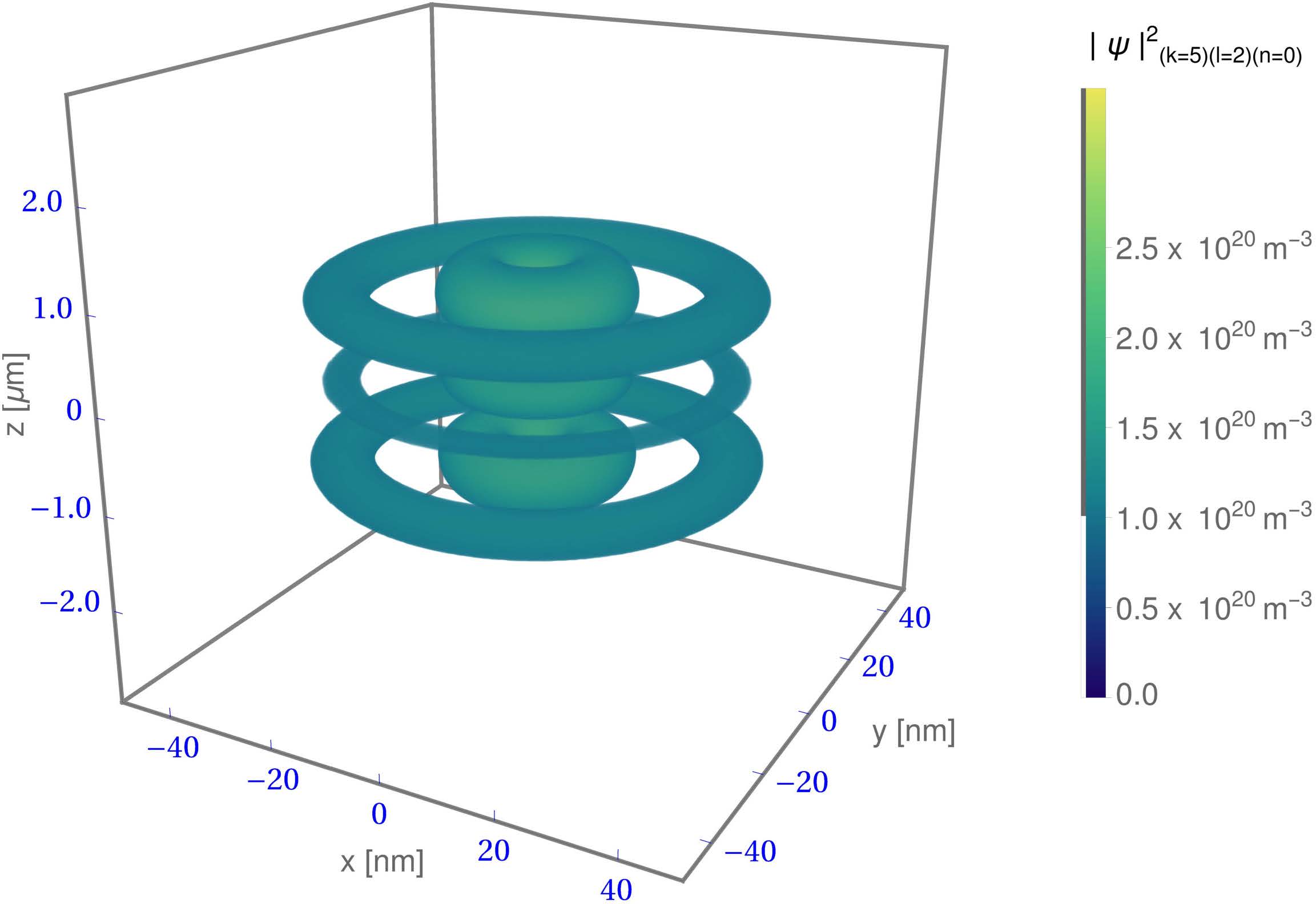
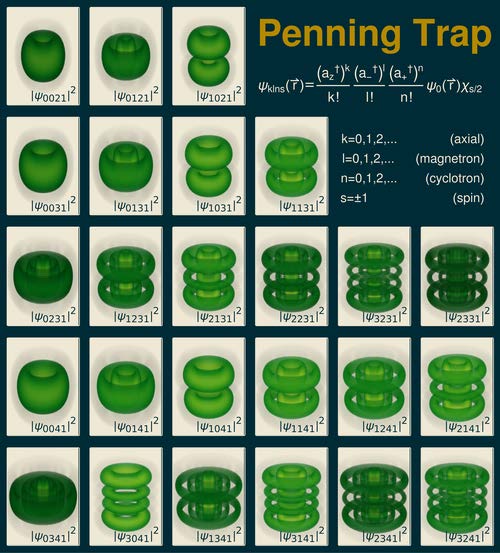
During the CFP Templeton Grant Period, we were able to extend the formalism of relativistic corrections to quantum cyclotron levels to sixth order in the momentum operators, i.e., sixth order in a a generalized “cyclotron coupling parameter”. Previous calculations extended fourth order in the momenta. To this end, the Foldy-Wouthuysen transformation needed to be generalized to higher orders, with careful account of the magnetic-field terms. The previously known fourth-order corrections were revisited on the occasion, and a small modification of previous results was found. Genuine QED corrections of the quantum cyclotron levels in the trap due to the changes in field quantization introduced by the boundary conditions induced by the walls of the cylindrical trap were also analyzed. It was found that the largest modification is received by the axial frequency, followed by the cyclotron and spin-flip frequencies.
- Limits to accuracy of g factor measurements in Penning traps due to boundary-induced effects.
- Re-analysis of existing results, and analysis of higher-order relativistic and quantum electrodynamic corrections, to quantum cyclotron energy levels in Penning traps.
- Chris Moore [postdoc]
- Albert Wienczek [postdoc]
- Ulrich Jentschura [faculty]
- One has improved the understanding of the so-called Foldy-Wouthuysen transformation in strong electric and magnetic fields in atoms. This transformation serves to disentangle the particle and antiparticle Hamiltonians (time evolution operators), which are otherwise unified in the so-called Dirac Hamiltonian (Dirac equation). The transformation is usually applied to atoms, where it is sufficient to consider the external fields only up to first order. In the case of an electron bound in a Penning trap, the magnetic field, which is otherwise considered as an external field, needs to be taken into account in higher orders.
- As a result of the calculations, we have obtained explicit results for the corrections of sixth order in the momenta for quantum cyclotron levels in a Penning trap. This corresponds to the third order in the magnetic field (B3); new operators had to be derived. A recalculation of the corrections of fourth order in the momenta reveals a small modification of a previously obtained result [see Eq. (91) of Ref. [9]].
- The understanding of the environment-induced corrections to the g factor has been improved. Among many competing claims [1, 2, 3, 4, 5] and counter-claims [6], the work [7] stands the test of time, and its approach could be used to analyze the problem. (Roughly speaking, some very subtle considerations regarding permissible gauges of the vector potentials make the paper [1] invalid.) Adopting and modifying the approach used in Ref. [7], it has been found, in a (yet) unpublished internal report, that important terms in the boundary-conditioninduced modification of the photon propagator had been left out in Ref.[7]. As a result, it was found that the environment-induced correction to the axial frequency is largest, followed by the cyclotron frequency and the spin-flip frequency. Estimates were provided in internal documents for an electron in a cubic trap, in an internal report.
Two papers are in the planning:
Paper in planning #1 (Internal Report #1): one, a complete analysis of the leading quantum electrodynamic correction to quantum cyclotron energy levels, i.e., of the “trapped Bethe logarithm”. (In the notation motivated above, this energy shift is proportional to the fifth power of the quantum cyclotron coupling parameter, i.e., to the power of B5=2, where B is the magnetic induction field of the trap.)
A more complex situation than anticipated has arisen. The dominant quantum electrodynamic effect is the so-called self energy of the bound electron, while so-called vacuumpolarization corrections vanish to a very good degree of approximation. It is generally known that the main difficulty in analyzing quantum electrodynamic self-energy shifts of electronic bound states lies in the presence of two distinct energy scales in the problem: one, the energy scale of the binding potential (in the 2 case of the quantum cyclotron, the binding energy in the combined uniform magnetic field, and the quadrupole electric field), and two, the rest mass energy scale of the electron. In the case of hydrogen and other “traditional” atomic bound systems, role of the trap fields is taken over by the binding Coulomb field. For the case of atomic systems, Richard Feynman acknowledged the difficulties in the matching of the high- and low-energy parts in footnote 13 on page 777 of Ref. [8]. In the case of the quantum cyclotron energy levels, a deeper analysis reveals that the evaluation of a so- called two-magnetic-vertex scattering amplitude becomes necessary; this is in contrast to Coulombic bound systems, where otherwise a so-called form-factor treatment is sufficient. A corresponding calculation is currently in progress; a preliminary draft which summarizes the findings obtained so far is attached to this report.
- Paper in planning #2 (Internal Report #2): The boundary-condition induced corrections to the quantum electrodynamic self-energy corrections (not a redundant formulation) have been analyzed during the project, and two internal reports have been completed. This subproject was the main concern of the project. The first report concerns the recalculation of an effect which was analyzed in Ref. [6]. This calculation concerned the energy levels of an electron, subject to a strong magnetic field, in the vicinity of a (perfectly) conducting wall. It was found that, among many treatments of the effect existent in the literature, the treatment conferred in Ref. [6] is the most convincing one. According to this analysis, which is confirmed, the dominant effect concerns the cyclotron frequency, not the spin-flip frequency. It was then claimed that, if one models a cubic trap to be composed of six conducting planes, then an estimate can be obtained for the order-of- magnitude of the boundary-induced shift of the QED shift of the quantum cyclotron energy levels. The main effects concern that axial frequency, and the shift of the cyclotron frequency due to the upper and lower plate of the trap cylinder. If one would like to go further, beyond the conducting wall, then a careful analysis of the modes of the cavity becomes necessary. For a rectangular cavity, a preliminary result has been obtained by the PI. This result is being included in the internal report.
Both papers are currently being kept on hold pending completion. The PI is working on both of them. One conclusion is that, on the accuracy level of 10−12 for the cyclotron frequency, one needs to analyze not only the order-of-magnitude of the boundary-induced effect, but also, the detailed structure of the trap modes, which depend on the trap material, i.e., on the dielectric function (see also the included draft, i.e., Internal Report #3).
A. Wienczek, C. Moore and U. D. Jentschura, “Foldy-Wouthuysen Transformation in Strong Magnetic Fields and Relativistic Corrections for Quantum Cyclotron Energy Levels”, Phys. Rev. A 106, 012816 (2022). The support from the CFP grant was properly acknowledged. This is Ref. [9].
The two papers mentioned under [4.] are being included as preprints. It is expected to submit Internal Report #1 and Internal Report #2 to international journals soon.
- G. Barton and H. Grotch, J. Phys. A 10, 1201 (1977).
- G. Barton and N. S. J. Fawcett, Phys. Rep. 170, 1 (1988).
- E. Fischbach and N. Nakagawa, Phys. Lett. B 149, 504 (1984).
- E. Fischbach and N. Nakagawa, Phys. Rev. D 30, 2356 (1984).
- K. Svozil, Phys. Rev. Lett. 54, 742 (1985).
- D. G. Boulware and L. S. Brown, Phys. Rev. Lett. 55, 133 (1985).
- D. G. Boulware, L. S. Brown, and T. Lee, Phys. Rev. D 32, 729 (1985).
- R. P. Feynman, Phys. Rev. 76, 769 (1949).
- A. Wienczek, C. Moore, and U. D. Jentschura, Phys. Rev. A 106, 012816 (2022).
- L. S. Brown and G. Gabrielse, Rev. Mod. Phys. 58, 233 (1986).
- X. Fan and G. Gabrielse, Phys. Rev. Lett. 126, 070402 (2021).
- X. Fan and G. Gabrielse, Phys. Rev. A 103, 022824 (2021).
© 2018 - Last Updated: 01/31/2023 - Disclaimer
